Functions: Fractional functions
Linear fractional functions
A linear fractional function is a function of the form
\[f(x)=\frac{\blue{a}x+\green{b}}{\purple{c}x+\orange{d}}\]
where #\blue{a}#, #\green{b}#, #\purple{c}# and #\orange{d}# are numbers and #x# is a variable.
The graph of a linear fractional function is a hyperbola with a vertical and horizontal asymptote.
We determine the vertical asymptote of a linear fractional function #f(x)=\frac{\blue{a}x+\green{b}}{\purple{c}x+\orange{d}}# by making the denominator#\purple{c}x+\orange{d}# equal to #0# and solving this equation.
Hence, for the vertical asymptote we find \[x=-\frac{\orange{d}}{\purple{c}}\]
The horizontal asymptote can be determined by noting that for very high values of #x# the numbers #\green{b}# and #\orange{d}# are negligible relative to the terms with #x#.
Hence, for the horizontal asymptote we have \[y=\frac{\blue{a}x}{\purple{c}x}=\frac{\blue{a}}{\purple{c}}\]
Take a look at the function #f(x)=\frac{\blue{2}x+\green{-3}}{\purple{4}x+\orange{2}}#
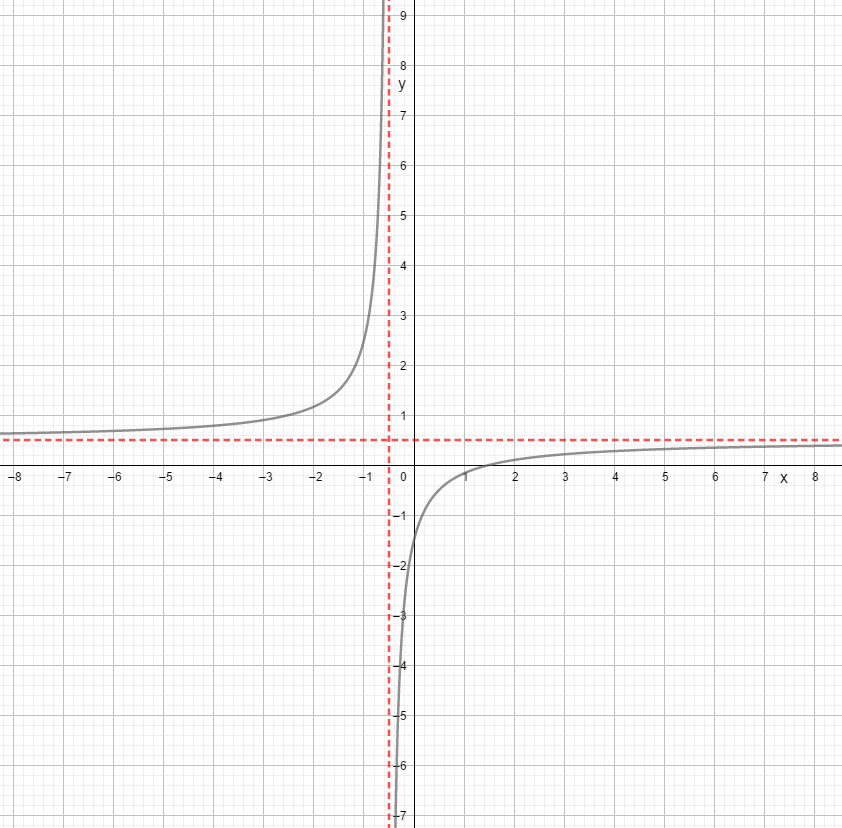
De verticale asymptoot is gelijk aan
\[x=-\frac{\orange{2}}{\purple{4}}=-\frac{1}{2}\]
De horizontale asymptoot is gelijk aan
\[y=\frac{\blue{2}}{\purple{4}}=\frac{1}{2}\]
Or visit omptest.org if jou are taking an OMPT exam.
