Growth rates and compound interest

Applications: Exponential functions and logarithms: Applications: Exponential functions and logarithms

Growth rates and compound interest

Exponential functions play an important role in many economic applications of mathematics. We have already seen the exponential function #f(x)=a^x#. Now, we will take a closer look at the exponential growth function, which is an exponential function multiplied by a constant #c#. This means that the value of #c# changes with the same percentage after each fixed length time interval. An example of such exponential growth is the interest on a savings account.

Exponential growth function

An exponential growth function has the function rule \[f(x) = \green{c} \cdot \
\blue{g}^x\]

Here,

\(\green{c}\) is the initial value, the function value at \(x=0\),
\(\blue{g}\) is the growth factor or the base,
\(x\) is the argument of the exponent

A growth that can be described by an exponential growth function, is also called an exponential growth model or simply exponential growth.

GeoGebra

Range
The exponential growth function is only defined for \(\blue{g} \gt 0\).

This affects the range of the exponential growth function:

if \(\green{c} \gt 0\), then \(f(x) \gt 0\) for all \(x\)
if \(\green{c} \lt 0\), then \(f(x) \lt 0\) for all \(x\)

with \(y=0\) as an asymptote for \(\blue{g} \neq 1\). This means that the function never assumes the value #0#, while the function values come arbitrarily close to it. The function value approaches #0# as #x# moves farther from #0#, and It is negative in the case #\blue{g}\gt 0# and positive in the case #\blue{g}\lt 0#.

If \(\green{c} = 0\), then #f(x)# is the constant function #0#; that is, \(f(x) = 0\) for all \(x\).

The growth factor in this exponential growth model indicates how quickly the function increases or decreases. We will now examine the connection between the growth factor and the growth rate.

Growth rate

The growth rate of exponential growth with function rule \[f(x) = \green{c} \cdot \blue{g}^x\]is equal to \(\blue{g}-1\).

If we multiply the growth rate by \(100\), we get the fixed percentage increase or decrease of the exponential growth model.

Example

Growth factor: #\blue{1.2}#

Growth rate: #0.2#

Percentage increase: #20\%#

Thus, the growth rate is the relative growth of the exponential growth model on an interval of length #1#.

Increase or decrease
As we have seen in the definition of the exponential function, whether there is an increase or decrease depends on the size of #\blue{g}# .

If \(\blue{g} \gt 1\), then \(f(x) \) increases.
If \(0 \lt \blue{g} \lt 1\), then \(f(x) \) decreases.

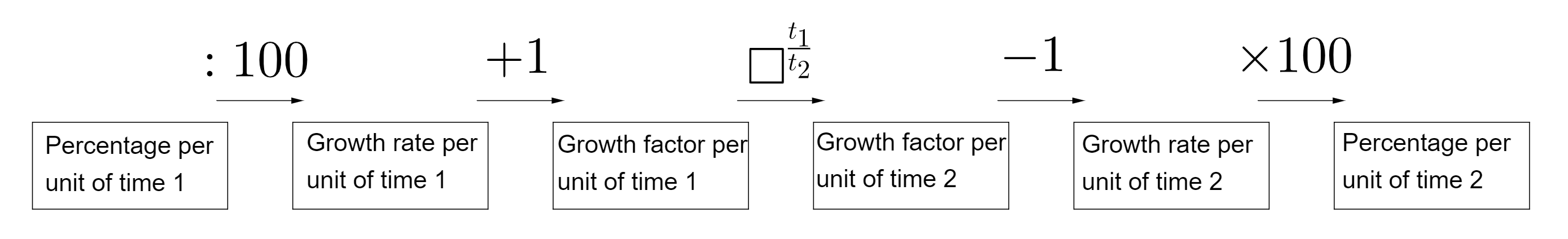
We often want to compare two exponential growth processes with different periods. For example, compare two savings accounts where the first savings account has a monthly interest rate and the second savings account has a yearly interest rate. The following theorem provides a formula to converse the growth factor in one time period to the growth factor in another time period.

Conversion formula for growth factors

Suppose that the growth factor per period #\orange{t_1}# is equal to #\blue{g}_1# and the growth factor per period #\purple{t_2}# is equal to #\blue{g}_2#.
Here, #\orange{t_1}# and #\purple{t_2}# are expressed in the same units of time.
Then \[ \blue{g}_2=\blue{g}_1^{\frac{\purple{t_2}}{\orange{t_1}}}\]

Example

If #\blue{g}_1=1.005# per #\orange{t_1}= \orange{\text{month}}#

then

#\blue{g}_2=1.005^{\frac{\purple{12}}{\orange{1}}}\approx 1.062# per #\purple{t_2}=\purple{\text{year}}#

Converting interest rates
To convert the interest rate in one unit of time to another, we first transform the interest rate to a growth factor. Then we adjust the growth factor to the new unit of time. Finally, we convert this new growth factor back into an interest rate. This procedure is illustrated by the following diagram:

The transformation of the growth percentage from a period of length #\orange{t_1}# to a period of length #\purple{t_2}# is expressed by the formula below, where #p_1# and #p_2# represent the growth percentages for #\orange{t_1}# and #\purple{t_2}#, respectively.

\[ p_2 = 100\cdot \left( \left(\dfrac{p_1}{100}+1\right)^{\frac{\purple{t_2}}{\orange{t_1}}}-1\right)\]

This formula is derived from the Conversion formula for growth factors using the equalities #\blue{g}_k = \frac{p_k}{100}+1# for #k=1# and #k=2#.

A special case of the exponential growth function is the formula for the future value without additional deposit. This formula is used when someone deposits an initial capital #S_0# in a bank over a number #n# periods (e.g., years) accruing compound interest at a certain rate per period. The formula gives the future value after the duration #n# if no additional money is deposited (nor withdrawn) in the interim. We describe these results in terms of the growth rate. The growth rate is determined by the interest rate.

Formula for future value without additional deposit

The future value #S(n)# in a compound interest scenario can be calculated with the function rule

\[ S(n)=\green{S_0}\cdot\left(\blue{1+i}\right)^n\]

Here,

#\green{S_0}# is the initial capital,
#n# is the number of periods,
#i# is the growth rate per period.

Example

After #5# years an initial capital of #\euro \, \green{5000}# euros with an interest rate of #2\%# is equal to
\[\begin{array}{rcl}S(5)&=&\green{5000} \cdot \left(\blue{1+\frac{2}{100}}\right)^5\\ \\&\approx& \euro \, 5520.40\end{array}\]

Since the capital is multiplied by the same factor each period, we are dealing with exponential growth. Therefore, the formula for #S(n)# represents the model for growth with growth factor #\blue{1+i}# and initial value #\green{S_0}#.

If the percentage is #p\%#, then the growth rate is #i=\frac{p}{100}#. The growth factor (the factor by which the capital at the beginning of the period must be multiplied in order to obtain the capital at the end of that period) is #\blue{1+i}=\blue{1+\frac{p}{100}}#.

Calculating other values
Not only can we use this formula to calculate the future value, but we can also determine the initial capital, the period #n# or the growth rate/interest rate with this formula using reduction.

Names and notation

Names and notations may differ in different sources. We will now summarise some of the notations and names you may encounter.

The growth rate is also called growth percentage or interest rate.

In Financial Mathematics, the factor #\left(\blue{1+i}\right)^n# is often denoted by #S_{\left.n\right \rceil i}#.

The future value is abbreviated as #FV#.

The initial capital #\green{S(0)}# is also called the present value or start value. The present value is abbreviated as #PV#.